3 things I've learnt:
1. Logic lies in the 'noun'.
Addition can only take place within items of the same noun, e.g. 1 flower and 4 flowers. One cannot add 1 flower and 2 pots- it does not make sense. Along the same line, when teaching fractions, avoid calling 3/4 'three out of/over four'. Instead, say 'three-fourths'. Why? Calling 3/4 'three-fourths' helps children to see it as a whole unit, and not as to separate units. This is necessary when the child learns to add fractions because then he who can add 1 flower to 4 flowers can add 'three-fourths' to 'one-fourth'. (The noun is significant because it aids logical understanding of the concept.)
The child who can add 1/2 to 2/4 and obtain 3/6 for his answer shows that he obviously does not understand the concept of fractions. One half and two fourths cannot add up because addition is only possible when the nouns of the addends are common!
2. A sound foundation in number bonds is a strategy.
How are number bonds- the very rudiments of Math education- useful knowledge for tackling bigger problems? Take this for instance: 9 x 5. If a child suddenly blanks out at this problem, he can summon his knowledge of number bonds to his aid. Number bonds will help him see that 9 is made up of, say, 4 and 5. And so if he knows the times tables for 4x5 and 5x5, finding out the answers for both and then adding them up can help him obtain the solution for 9x5. Alternatively, he can use 3x5 and 6x5 as well. Helping a child gain a strong foundation in number bonds is equipping him with a strategy that he can use in solving difficult problems in future math exercises.
3. The beginning of 'Measurement'.
The first step to mastering measurement is to teach children to compare lengths, sizes, weight, etc. Comparing comes before measuring. There are two stages in getting children to do comparisons. The first stage is 'direct comparison'- when two objects are placed side by side and are compared that way. After this is established, the teacher and learner can then go on to 'indirect comparison'- when the objects compared are objects that cannot be brought together (e.g. the length of a whale vs the length of a sea lion).
Once indirect comparison is established, children may begin measuring with non-standard units. Then again, there are also 2 stages in this phase. The first phase is to measure with many non-standard units e.g. measuring the length of a shoe with paperclips. Once that is understood, the second phase is then to measure with one non-standard unit e.g. how many toothpicks long is this table?
Some children are unable to reconcile or associate the number of non-standard units with the length of the object measured. They get confused thinking that counting the number of units is a separate activity from measuring the length of the object and are not able to make the connection between the number of units and the length. The way to help them make that association is to ensure that the language that we as teachers use when verbalizing the measurements, is complete. So for instance we can say, "Yes, the length of this table is 12 toothpicks long," or "This table is as long as 12 toothpicks." As we take note to always say the number of the non-standard unit with the length of the object measured, children will learn to associate the number of units with the length over time.
2 Questions I have:
1. If the society is headed so stubbornly in the direction of 'accelerated' learning, won't 'enrichment' learning give my child less of a competitive edge amongst his peers, albeit him understanding each topic taught more thoroughly?
2. Using strategies such as number bonds and breaking problems down into manageable portions help a child to tackle problems more ably. However, it may also result in a slower working process. When is a child expected to be able to use more efficient methods to solve problems?
I Gotta Love Math!
Tuesday, 24 July 2012
Monday, 23 July 2012
Teaching Math with Technology
I am all for the idea. Math teaching and learning can be brought to a whole new level with the use of technology. I love how this teacher in the video used technology as a tool to reach out to his students, help them visualize Math concepts better, AND as a classroom management tool as well!
The Math teacher here was seen using a projector to enhance his teaching. If he tried to demonstrate a concept with these little tangram pieces without the projector, his students sitting at the back of the class would probably be at the disadvantage of not being able to see his demonstration very well. Instead, by using the projector to enlarge the demonstration and inviting a student to help him with the demonstration while he explains the concept, the teacher does two things: He reaches out to the WHOLE class while teaching, and fosters active learning with the child at the projector as well as the rest of his class who are able to follow his instruction closely. By using technology in the classroom, the children are also observing the teacher use the technology tools and gaining tech savvy themselves in the process.
Technology can help to enhance hands-on learning in the classroom. For instance, children who use technological tools to solve or present their work have to make independent decisions about how to manipulate the tools or the problem, the format in which they are going to present their work; and when activities are conducted and presentation time follows, it is more effective and efficient for children to 'show-and-tell' on their work through displaying it to the class through the projector than it is for everyone to walk around the class to examine each other's work. Also, if a child has a question, the teacher can use his technology tool to share the problem with the class to call for collective problem-solving and various solutions.
With technology as the mode of instruction, a teacher's role also changes. The focus is now on the subject presented via the technological tool, as well as the tool as the instructor. The teacher takes a back seat and becomes a facilitator who peers over children's shoulders to check on their learning process, intervening only when necessary.With the present craze over in technological gadgets among the youth today, integrating technology into education means fostering greater interest in learning. This greater interest in learning may also mean better attitudes toward subjects taught, and better results.
Technological tools are set in this day and age to replace the old-school textbooks and paper-and-pencil style of work. With technology, work can be done faster, time spent more efficiently, and children are willing to do more if that means they will be using the technological tool to work. Using technology to teach Math may also be a means of reaching out to some learners who just can't seem to take to the conventional 'worksheet' or 'exercise book' style of math practice, and can help students with visualizing difficult concepts.
The only dampener in this whole discussion is the reality of lack of resources in some of our schools and childcare centers to use technological support to enhance teaching and learning. If the benefits of using technology to teach could be proved to all, perhaps institutions would be more willing to invest in these tools which will bring educational improvements in almost every way across the board.
Prepare Our Children.
"Preschool is about preparing children to learn, e.g. we prepare children to learn addition, subtraction; not teaching them the concept yet. It's about preparing the child to handle the content." -Dr Yeap, 19 July 2012
When Dr Yeap made this point, I pricked up my ears. So as an early childhood educator, my job is to prepare the children to handle the content of higher education. How? How can I prepare a child's mind for the demands of higher mathematics- multiplication, division, fractions- besides by infusing his milk with plenty of DHA, making sure he gets his daily dose of omega three fatty acids, ensuring he gets a solid breakfast in the mornings so his brain is fueled and powered-up to go, and employing a tutor to teach him the basics of these concepts ahead of the school syllabus?
The answer comes in the form of a math problem:
Find a way to turn this trapezoid into a rectangle with the same area.
After giving us some time to figure it out and putting up a few of the possible solutions some of us figured out, Mr Yeap used the following example to convey his point:
When Dr Yeap made this point, I pricked up my ears. So as an early childhood educator, my job is to prepare the children to handle the content of higher education. How? How can I prepare a child's mind for the demands of higher mathematics- multiplication, division, fractions- besides by infusing his milk with plenty of DHA, making sure he gets his daily dose of omega three fatty acids, ensuring he gets a solid breakfast in the mornings so his brain is fueled and powered-up to go, and employing a tutor to teach him the basics of these concepts ahead of the school syllabus?
The answer comes in the form of a math problem:
Find a way to turn this trapezoid into a rectangle with the same area.
 |
| Cut the trapezoid in half this way |
 |
| Place the pieces together this way |
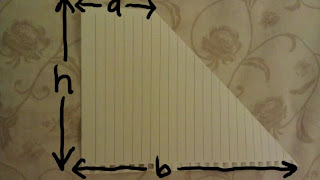
After the pieces were placed together to form a rectangle, Mr Yeap then asked us if we could find the area of the rectangle based on the measurements provided for the trapezoid, and how we could do it. Seeing that sides 'a' and 'b' of the trapezoid were still the same in the rectangle, we quickly identified them. The height of the trapezoid, 'h', was halved to form the rectangle and thus we derived the breath of the rectangle as '1/2h'. Therefore, the area of the trapezoid was 1/2h(a+b), based on the area of the rectangle. And isn't this very formula, Mr Yeap pointed out, the formula for finding the area of a trapezoid that we were taught back in school? I had my EUREKA! moment right there and then. YES IT WAS AND OH NOW I SEE, that's how it is derived! I was totally converted to 'Yeap-emathetics'. This is what visualization is all about- being able to see things that are difficult to see, learning to view a problems from multiple perspectives. This is the strategy of using prior knowledge to figure out solutions to problems- in action! Children try to adjust problems into manageable parts and then use what they already know to solve them. This is also how Math becomes understood.
This is what it means by preparing childrens' minds to learn and to extend their own learning: giving them practice in breaking down problems into simpler portions that they can manage with the knowledge that they have. Instead of blindly committing working and solutions to memory which leaves children stumped when they encounter challenges, we teach them the strategy of adopting multiple perspectives which will help them to attempt these problems and to develop a 'can-do' and'will-try' attitude in any challenge they encounter in life.
Friday, 20 July 2012
Okay that's true.
"Research indicates that early number sense predicts school success more than other measures of cognition like verbal, spatial, or memory skills or reading ability." (Pg 171 of text)
I am about to jump at that audacious statement with my claws out at the ready, poised to maul it to pieces. Does it mean to say that the mastery of literacy (*sensitive subject*) is a lesser ability than the mastery of number sense?? What an insult! I take serious offence with this statement.
WELL. To that, Mr Yeap had this to say (and I paraphrase):
"Why must we train children to solve "How many 1/2s are there in 3/4"? Is it so that they can use it in the supermarket? No! Teaching them strategies to solve this problem will train their brains to look for things that are difficult to see. This is called the ability to 'visualize' and it is so important in the later stages of learning. Learning to 'visualise' teaches children to answer inferential questions, to read in between the lines- skills that will serve them well in other subjects such as literature and science."
Alright, that's true, but I still don't like how it makes literacy sound less important in comparison.
#pardontheliteracystudent
Basic Fact Mastery. How did that happen? (Chapter 10)
Chapter 10 is all about helping students to get their 'basic facts' straight. By the term 'basic facts', the authors mean the ability to give a quick response (in 3 seconds) to an equation without having to resort to inefficient means to calculate the solution, e.g. counting by ones. When a child is savvy with basic facts, he can more easily do mental calculations through numerical reasoning in his head. He is not reliant on calculators or inefficient working-methods to do simple computations.
This is an interesting topic. Come to think about it, when and how did you and I ever get to the point of just simply knowing that 5x6=30 or that 8+6=14, without needing to count or to even think about it? It's a curious thing. I can't seem to pinpoint a specific time when it happened to me; it seemed to be a cumulative, gradual experience borne of consistent exposure to these combinations and practice in computing such sums.
(My timing for the above exercise is 26secs, FYI.)
My husband told me that when he was little, his dad would often create exercises like this for the kids to work on; the goal, he said, was to beat each other's timings and to train their brains to be able to answer these simple sums instantly by recognition of/familiarity with the combinations of numbers. I wonder how productive such 'drills' are.
Well, I've just found my answer to that and I'm going to just quote and unquote it in a chunk:
"You may be tempted to respond that you learned your facts in this manner, as did many other students. However, studies...concluded that students develop a variety of different thought processes or strategies for basic facts in spite of the amount of isolated drill they experience. Unfortunately, drill does not encourage or support the refinement of these strategies...this approach to basic fact instructions works against the development of...mathematics proficiency..." (pg 173 of the text; italics added for emphasis)
It is so true, the fact the memorization is but a quick fix to Math problems . But what happens when memory fails you? Memorization leaves you with zilch strategies to resolve the difficulty- it fails you too (memorizing isolated facts without a basis or context is worse). It is unfortunate that I am a product of such drills. I could have been very successful at using strategies if only I'd been taught them when my brain was still young and quick; not so sluggish and set in its stubborn old ways as it is now. However, I am hopeful about re-learning my fundamentals in mathematics as I teach it to my children. It's never too late!
Chapter 10 is an amazing collection of methods that educators can use to guide children in strategy development in addition, subtraction, multiplication and division- towards attaining basic fact mastery. If you want your child to attain mathematics proficiency and have a happier time while learning and doing math, please take note of this chapter?
References:
Van de Walle, J. Elementary and middle school mathematics: Teaching developmentally (8th Edition). New York: Longman.
Try this out for fun:
This is an interesting topic. Come to think about it, when and how did you and I ever get to the point of just simply knowing that 5x6=30 or that 8+6=14, without needing to count or to even think about it? It's a curious thing. I can't seem to pinpoint a specific time when it happened to me; it seemed to be a cumulative, gradual experience borne of consistent exposure to these combinations and practice in computing such sums.
(My timing for the above exercise is 26secs, FYI.)
Apparently, there are 3 phases to development of basic fact mastery, according to Baroody, a mathematician who researches basic fact mastery (Pg 172 of the text):
Phase 1- Counting strategies (using fingers or objects to count verbally to get the answer)
Phase 2- Reasoning strategies (using known information to logically determine an unknown combination)
Phase 3- Mastery (producing answers efficiently, quickly, and accurately)
My husband told me that when he was little, his dad would often create exercises like this for the kids to work on; the goal, he said, was to beat each other's timings and to train their brains to be able to answer these simple sums instantly by recognition of/familiarity with the combinations of numbers. I wonder how productive such 'drills' are.
Well, I've just found my answer to that and I'm going to just quote and unquote it in a chunk:
"You may be tempted to respond that you learned your facts in this manner, as did many other students. However, studies...concluded that students develop a variety of different thought processes or strategies for basic facts in spite of the amount of isolated drill they experience. Unfortunately, drill does not encourage or support the refinement of these strategies...this approach to basic fact instructions works against the development of...mathematics proficiency..." (pg 173 of the text; italics added for emphasis)
It is so true, the fact the memorization is but a quick fix to Math problems . But what happens when memory fails you? Memorization leaves you with zilch strategies to resolve the difficulty- it fails you too (memorizing isolated facts without a basis or context is worse). It is unfortunate that I am a product of such drills. I could have been very successful at using strategies if only I'd been taught them when my brain was still young and quick; not so sluggish and set in its stubborn old ways as it is now. However, I am hopeful about re-learning my fundamentals in mathematics as I teach it to my children. It's never too late!
Chapter 10 is an amazing collection of methods that educators can use to guide children in strategy development in addition, subtraction, multiplication and division- towards attaining basic fact mastery. If you want your child to attain mathematics proficiency and have a happier time while learning and doing math, please take note of this chapter?
References:
Van de Walle, J. Elementary and middle school mathematics: Teaching developmentally (8th Edition). New York: Longman.
Thursday, 19 July 2012
Monday at Elementary Mathematics
So what I found interesting about the first session of class was how Mr Yeap used a magic trick with poker cards to teach math concepts! I thought the idea was unconventional (no doubt suggestive and might send questioning parents over in a flurry), creative, and contained a multifaceted lesson on patterns, multiples, and ordinal numbers. Activities like this open up various doors and opportunities for learning as they are open-ended and offer multiple avenues for discovery. I also learnt that children learn Math through creative and concrete means prepared thoughtfully by the teacher (yes, a rationale lies behind every decision- every choice of words, numbers, questions- made throughout the preparation and teaching process).
I also appreciated how My Yeap let us struggle through each new activity within our groups before providing us with the answer. I found it very helpful to be able to interact with my friends while tackling the problem because I found myself learning a lot- mostly, in fact- from them! I experienced Lev Vygotsky's sociocultural theory for myself: I went through states of disequilibrium, equilibrium, assimilation and accommodation; I was scaffolded by my peers and teacher within my ZPD; I was learning through social interaction!
After giving us some time to work the problems out on our own, Mr Yeap would then call out for the different solutions that we used to solve the problems. He was quick to point out that there are various approaches to solving a problem- not just one hard and fast rule. I really appreciated that. Even if the solution I used was not as efficient as another's, it was still a valid, legitimate one and being assured of that made me feel capable.
I also appreciated how My Yeap let us struggle through each new activity within our groups before providing us with the answer. I found it very helpful to be able to interact with my friends while tackling the problem because I found myself learning a lot- mostly, in fact- from them! I experienced Lev Vygotsky's sociocultural theory for myself: I went through states of disequilibrium, equilibrium, assimilation and accommodation; I was scaffolded by my peers and teacher within my ZPD; I was learning through social interaction!
After giving us some time to work the problems out on our own, Mr Yeap would then call out for the different solutions that we used to solve the problems. He was quick to point out that there are various approaches to solving a problem- not just one hard and fast rule. I really appreciated that. Even if the solution I used was not as efficient as another's, it was still a valid, legitimate one and being assured of that made me feel capable.
Sunday, 15 July 2012
Chapter 2
I appreciate the sections on 'Implications for Teaching Mathematics' and 'Relational Understanding', leading to 'Mathematics Proficiency' and 'Benefits of Developing Mathematics Proficiency' in Chapter Two of the textbook. I feel that I was unfortunate to have functioned largely on instrumental understanding while doing Math throughout my years of schooling, and I am very interested to learn how to teach preschoolers- to set the foundation right early in their lives- to achieve relational understanding in the subject, and toward attaining mathematics proficiency.
I was fortunate to have a great Math teacher in my final year in Secondary school as I was preparing for the GCE 'O' Levels. Mr Lee Han Seng was a serious, stern, no-nonsense kind of man, but he had a gift for dissecting complicated Math concepts into digestible portions and presenting them very simply and explaining them very systematically, logically and comprehensibly. He developed in me a liking for the subject and he made me see that I had an aptitude for it if only I would change my attitude toward it and be willing to give my all to understand and to practice consistently. Looking back, I was sure Mr Lee did his own 'homework' before stepping into class everyday. I am very sure he thought hard about what was the best way to present the math concept for the day; how to explain it and build up our understanding step by step, to the point where we could comprehend what the math problem exacted of us.
My Math tutors in the past have all at some point, asked me this same question while I was tackling a problem sum: "Can you tell me why you have to divide/multiply/add/subtract this by this? Do you know why you have to do it or are you just guessing?"" Truth be told, it was purely a guessing exercise for me 70% of the time. Looking back, I feel that it is indeed crucial to understand Math! It is not a rote memorization-then-regurgitation exercise. Math has meaning. A teacher can tell whether a child has gained proficiency in a Math exercise by requiring that and listening to the child to explain the steps/workings he does to solve the problem. To be able to teach Math such that my students will be able to acquire such understanding and proficiency is really my biggest goal for this module, because really, to understand math is the only way to attaining math proficiency.
The text talked about engaging students in a productive struggle- giving them time to struggle through the mathematical concepts they are exploring. I feel that such a 'struggle' can only be productive if the student has his/her foundational concepts in Math already set in place; only then can new knowledge be built upon prior knowledge. Otherwise, most times, students are just staring blankly at a question, too afraid to make a wrong move, and too afraid to admit that he/she does not know how to attempt the question, and thus, are just sitting there, wasting time. New content has to be scaffolded for less competent math learners.
At this point I wish I had preserved some of the worksheets or past exam papers containing math problems I had attempted carelessly or thoughtlessly and show how I was made to do corrections, but not with understanding either, to be examined and to re-attempt understanding and doing them now! Unfortunately I had already discarded the lot of them upon graduation from secondary school. I am not a competent Math student, but I am very interested in finding out how this module can transform me into a competent Math teacher!
I was fortunate to have a great Math teacher in my final year in Secondary school as I was preparing for the GCE 'O' Levels. Mr Lee Han Seng was a serious, stern, no-nonsense kind of man, but he had a gift for dissecting complicated Math concepts into digestible portions and presenting them very simply and explaining them very systematically, logically and comprehensibly. He developed in me a liking for the subject and he made me see that I had an aptitude for it if only I would change my attitude toward it and be willing to give my all to understand and to practice consistently. Looking back, I was sure Mr Lee did his own 'homework' before stepping into class everyday. I am very sure he thought hard about what was the best way to present the math concept for the day; how to explain it and build up our understanding step by step, to the point where we could comprehend what the math problem exacted of us.
My Math tutors in the past have all at some point, asked me this same question while I was tackling a problem sum: "Can you tell me why you have to divide/multiply/add/subtract this by this? Do you know why you have to do it or are you just guessing?"" Truth be told, it was purely a guessing exercise for me 70% of the time. Looking back, I feel that it is indeed crucial to understand Math! It is not a rote memorization-then-regurgitation exercise. Math has meaning. A teacher can tell whether a child has gained proficiency in a Math exercise by requiring that and listening to the child to explain the steps/workings he does to solve the problem. To be able to teach Math such that my students will be able to acquire such understanding and proficiency is really my biggest goal for this module, because really, to understand math is the only way to attaining math proficiency.
The text talked about engaging students in a productive struggle- giving them time to struggle through the mathematical concepts they are exploring. I feel that such a 'struggle' can only be productive if the student has his/her foundational concepts in Math already set in place; only then can new knowledge be built upon prior knowledge. Otherwise, most times, students are just staring blankly at a question, too afraid to make a wrong move, and too afraid to admit that he/she does not know how to attempt the question, and thus, are just sitting there, wasting time. New content has to be scaffolded for less competent math learners.
At this point I wish I had preserved some of the worksheets or past exam papers containing math problems I had attempted carelessly or thoughtlessly and show how I was made to do corrections, but not with understanding either, to be examined and to re-attempt understanding and doing them now! Unfortunately I had already discarded the lot of them upon graduation from secondary school. I am not a competent Math student, but I am very interested in finding out how this module can transform me into a competent Math teacher!
Subscribe to:
Posts (Atom)